This series of worksheets and lessons will help students learn the basic concept of the commutative property as it relates to addition operations. In essence this property of addition says that when you have an addition equation, the order of the addends does not matter. Meaning that the overall sum is not changed on bit. In as simple an example as possible: 1 + 2 = 3 and also 2 + 1 = 3. Whether 2 or 1 comes first or second does not change anything. Below you will find worksheets that will help make this concept concrete for students.
Print Commutative Property of Addition Worksheets
Click the buttons to print each worksheet and associated answer key.

Rewrite Using Commutative Property Lesson
This worksheet explains how to rewrite equations using the commutative properties of addition. A sample problem is solved and then you solve 2 problems of the same nature on your own.

Rewriting Lesson and Practice
Students will rewrite equations using the commutative properties. A sample problem is solved and two practice problems are provided.

Rewriting Worksheet
Restate all these expressions and equations based on what we have learned. Ten problems are provided.


Commutative Property Drill
Students develop their skills in rewriting equations using the commutative property. Eight problems are provided.
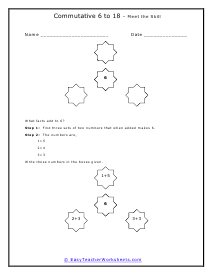
Making a Sum of 6 Lesson
Find three sets of two numbers that when added makes a sum of 6. Write these numbers in the boxes that are given.
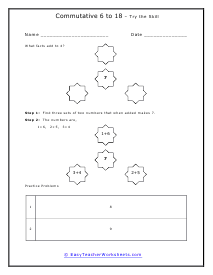

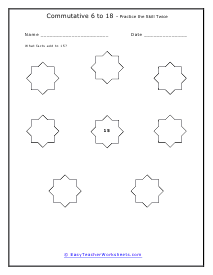


Math Facts of 18
This is the mega bomb of a problem for most younger students, but they can get it done.

Commutative Property Warm Up
Get yourself together with this group activity. Three problems are provided.
What is the Commutative Property of Addition?
Mathematics is a tricky subject and has a lot of different properties of numbers through which new formulae and identities are formed. There are many number properties to which arithmetic operations are applied. In this post, we will talk about the commutative property of addition.
Commutative" comes from the word "commute", which means to travel about. This is exactly what is achieved through the standard way we go about adding of terms. The general formula that is often used to express this is a + b = b + a. It basically means that terms can float around all they want, but addition is addition. A sum is a sum. When you see terms dancing around is an example of the Commutative Property. This property really helps us in algebra to restate terms and help organize equations. In a nutshell, the terms in any operation undergoing solely addition can be placed in any order of your choice. The outcome of the sum will always be the same and there is nothing to worry about here. For example, if we were to add the following four integers: 7, 8, 2, and 4. We could write this 7 + 8 + 2 + 4 or any combination we so choose. 7 + 8 + 2 + 4 will result in a final outcome of 21. So will 4 + 8 + 2 +7. Just because we have changed the order in which the integers were presented, the final value is never changed. This can also be applied to multiplication and you will see that topic come up more as we move through this.
If we move the operands around, and if that does not affect the arithmetic operation and the answer in the equation, then the arithmetic operation is said to have commutative property.
For example, if there are two numbers, X and Y, then the formula of the commutative property of numbers is shown as,
X + Y = Y + X
X × Y = Y × X
X - Y ≠ Y - X
X ÷ Y ≠ Y ÷ X
The commutative property is true for multiplication (3 x 2 = 2 x 3) and addition (3 + 2 = 2 + 3). However, it is not true for the subtraction (3 - 2 ≠ 2 - 3) and division (3/2 ≠ 2/3). This shows that order does not matter in addition or multiplication, but for subtraction or division, it changes the answer.
Let's learn with examples the commutative property of addition in detail. The commutative property of addition says that the order of numbers does not change the result of the sum.
The commutative property is shown as A + B = B + A.
The numbers A and B are called addends, and the equation is called a sum.
Examples
To understand the property in detail, let's learn from the application of the property. For example, 8 + 7 = 7 + 8 = 15. Here adding 7 to 8 or 8 to 7 gives us the same result.
If two numbers are given 12 and 13, then 12 + 13 = 25 and 13 + 12 = 25, answer for both is 25.
Using brackets won't affect the property or the answer, such as: (2 + 5) + 8 = 15 vs. (8 + 5) + 2 = 15. Both the answers are the same here. However, this is another property known as the associative property of addition which states that if the grouping is changed for the addends, it won't change the answer.
To embed the things we learned today, why not try some word problems for the commutative property of addition.
Example: Anna had 6green apples in her shopping cart. She met Ryan, who gave her 5red apples. How many apples does Anna have at the end?
Now, adding 6 and 5 in any order will give the same answer because, in the end, Anna will have a total of 11 apples.
Try It Out Yourself!
Question 1: Adriana is stringing 3 red, 4 green, and 2 yellow beads to make a bracelet. Will changing the order of the beads change the total?
Question 2: To start the construction of a building, Carl and his partner needed to gather some wood from the forest. If they initially had 13 extra planks of wood in the house and Carl and his partner got 10 planks of wood each, how many pieces of wood do they have in total?
Recap
Here is a short recap of what we learned.
A + B = B + A.
The commutative property of addition states that the order of numbers while addition does not change the answer. However, it matters when two numbers are subtracted or divided.
How to Rewrite Addition Problems Using the Commutative Property
You can change the order of the multiplied and added numbers by rewriting expressions by a commutative property. ÷Commutative Properties - With respect to addition - When a and b are real numbers, the formula will be; a + b = b + a. With respect to multiplication - When a and b are real numbers, the formula will be; a × b = a × b.
The commutative property also applies to must product-based equations. But that is an entirely different lesson. While this concept may seem obvious to you, it is helpful to keep in mind as we leap into algebra. Sometimes we lose sight of the basic concepts of math when we move away from integers and towards variables that we will often see in algebra. This concept will also have a great deal of impact in studies with geometry. It kind of spans all main branches of math and will help you immensely as you make your way across the curriculum.
How to use the Commutative Properties - You will get the same numbers by adding these numbers separately; 5 + 3 = 8 | 3 + 5 = 8. Here, you don't need to be anxious about the correct order of the numbers. You will apply the same method while multiplying 5 and 3. 5 × 3 = 15 | 5 × 3 = 15. The correct order of the numbers matters while solving the problems for commutative properties. The answer will be the same when you add or multiply the expressions by changing the correct order.
Rewriting the Equations - We have to use the commutative properties to rewrite the following equations; -1 + 3 = - 1 + 3 = | 4.9 = 4.9 = .
Solution - We will use the commutative property with respect to addition to change the order. -1 + 3 = | -1 + 3 = 3 + ( - 1 ). Also, we will use here commutative property with respect to multiplication to change the order. 4.9 = | 4.9 = 4.9.


