A set is basically a collection of data (numbers that represent values). When we talk about "building" a set, we are referring to designing a way to describe the set. These worksheets work on how to build sets with the proper interval notation. This skill will help you be able to better analyze data and share work with others. Students will be asked to convert algebraic inequalities into interval notation, identify differences in line graphs, write statements of given conditions, and more. Using these worksheets and lessons, your students will learn how to understand terms and concepts related to set builder and interval notation, and they will practice these skills by answering various questions related to the subject.
Print Set Builder and Interval Notation Worksheets
Click the buttons to print each worksheet and associated answer key.

Set Builder and Interval Notation Lesson
Learn about the different terms related to interval notation, and practice writing expressions as intervals. This lesson begins by having you interpret interval notation and then make your own over the course of 2 problems.

Set Builder and Interval Notation Worksheet 1
Using the information provided, answer the questions regarding interval notation. Example: Write the following inequality in interval notation: -6 < x < 3 or 5 > x > 7. If you can answer these questions confidently, you are in really good shape with this skill.
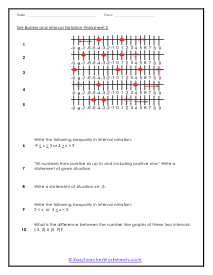
Practice Worksheet 2
Using the information provided, answer the questions regarding interval notation. Example: Write a statement of situation x ≠ -3. Students will have difficulty with understanding this statement at first.

Review Sheet
Review interval notation by following the review steps, then practice using the questions provided below. Example: What is the difference between the number line graphs of these two intervals: [-7, 0] U (4, 8]?

Quiz Sheet
Answer each question regarding interval notation, and then check your answers and record your total score below. Example: "All numbers from positive three up to and including positive two." Write a statement of given situation.

Do Now
All of these problems will ask you to create math statements and expressions that communicate some message. Here is an example: Write a statement of situation x ≠ 5.
What is Set and Interval Notation?
There are plenty of times that we need to express the concept of an inequality. Things like speed and weight limits fall into this category of mathematical expression. We started by visualizing inequalities on a number line and then we began to use graphs. This brings us to another method of describing inequalities and provide a medium that allows us to solve problems that include them.
A set is a mathematical concept for a collection of diverse things; they have elements or members that may be any mathematical object: lines, numbers, variables, points in space, symbols, and even geometrical structures.
In mathematics, a set is a logically arranged group of items that can be represented in either set builder or roster form.
They are typically denoted by curly brackets {}; for instance, A = {1, 2, 3, 4} is a set. They are also created to define the object collection.
A person represents the way sets are always the same: as a group of clearly defined objects or elements. A capital letter normally designates a set.
Examples Include
A = {1, 2, 3, 4, 5}
Since a capital letter is typically used to denote a set, as a result, A is the set, while 1, 2, 3, 4, and 5 are its components or individuals. The written items in the set may be arranged in any sequence, but they cannot be repeated. In the case of letters, each component of the set is represented by a little letter. Additionally, it can be written as 1 ∈ A, 2 ∈ A, etc. The set's cardinal number is 5.
The cardinal number is the number of elements in the specific set. In mathematics, an interval is a collection of real numbers that includes all real numbers that fall within any two of the set's numbers. Subsets of the real number line can be written using interval notation.
An interval is said to be closed if its endpoints are included, and in case it contains endpoints, it is known as an open interval. We use parentheses for open intervals, whereas closed brackets are utilized for closed intervals.
You may also write subsets of the number line composed of many intervals using interval notation and the set union operator.
As an illustration, the interval containing the integers 0, 1, and all other numbers is the set of numbers x satisfying 0 x 1.
Other instances of intervals are the set of integers such that 0 x 1, the set of all real numbers, the empty set, any singleton, the set of nonnegative real numbers, the set of positive real numbers, and the set of all real numbers will be displayed.
Formula
The formula to denote class interval is Upper-Class Limit-Lower-Class Limit.
In statistics, the data is divided into many classes, and the distance between classes is referred to as a class interval.
Solution
The number line's left to the right location in the solution is indicated using interval notation. Endpoints part of the solution are denoted by parentheses, while those not denoted by brackets.
Range
The interval notation is one instance of this. A real number interval is a set of real numbers between a and b in a b that includes all real numbers between the given start and end points, a and b. This notation is often used in Algebra and other complex math when representing an interval.
Points
Parentheses or rectangular brackets may be used to write intervals, while a comma is used to separate two numbers in mathematical expressions. The two values in question make up what is known as the endpoints of the interval. The number on the left side of the expression represents the lowest possible element. The number on the right is the most significant component, often known as the upper bound.


