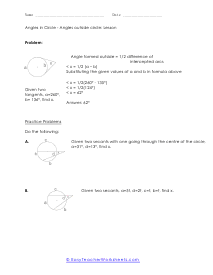
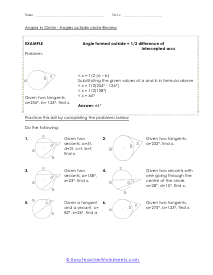
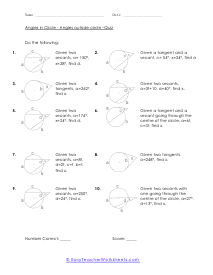
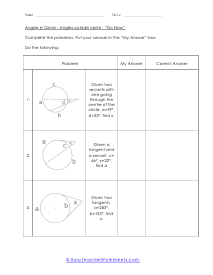
If you have a circle you can determine measure of the angle formed by two secants, tangents or a secant and a tangent from a point outside the circle using the outside angle theorem. The measure of that angle will be half of difference of the values of the intercepted arcs. We can use this for all sorts of rays. Scientists often use this theorem to understand the angle at which the sun light/heat rays touch Earth. If you think about it, the sun and the rays it gives off are just a circle and two tangent rays coming off of it.
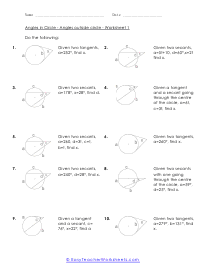
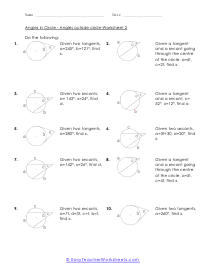
These worksheets explain how find the measure of an angle outside of a circle. Your students will use these worksheets to learn how to measure angles outside a circle, whether formed by tangents, secants, etc.