A relation is a relationship between sets of numbers. Relation can be expressed as the x-values and y-values of ordered pairs. Relationships in probability and statistics can take on many different forms. A statistical relationship is a blend of deterministic and unexpected links. A deterministic relationship includes a definite correlation among two variables. Let's suppose you earn $10 in one hour. For each hour you serve, you win an extra ten dollars. An unexpected alliance is a part of a misnomer because there is no link between the variables. However, probable methods may make it look like there is a relationship. For instance, you pay $20 on lottery coupons and get 25 Dollars. That earning is the part of coincidence, but it could let you believe that for every 20 dollars you pay for vouchers, you will win $25 more (which is incorrect). A statistical relationship is a blend of the above two loops. It is a bond that's a bit deterministic and part chance. In case, there is a statistical tie between calorie input and weight accrual. But the connection is not accurate: how much load you increase relies on different factors and some unexpected components. Does that 200 calorie Cadbury bar carry 200 calories? Did you have one bite of ice cream?
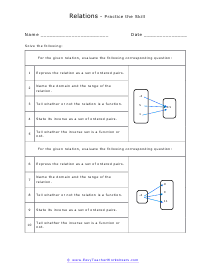
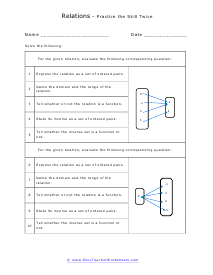
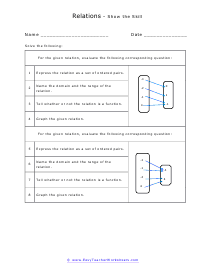
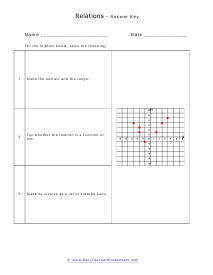
In these worksheets, students will work with relations. They will learn how to express a given relation as a set of ordered pairs. They will graph relations and draw mapping for the relations. Students will refer to diagrams as they solve problems. This set of worksheets contains lessons, step-by-step solutions to sample problems, and both simple and more complex problems. It also includes ample worksheets for students to practice independently. When finished with this set of worksheets, students will be able to express relation as a set of ordered pairs, and will be able to graph relations and draw maps for the relations. These worksheets explain how to interpret and present relations as ordered pairs, graphs, or diagrams. Sample problems are solved and practice problems are provided.